
The simplest descriptions of diffraction are those in which the situation can be reduced to a two-dimensional problem.

Usually, it is sufficient to determine these minima and maxima to explain the observed diffraction effects.
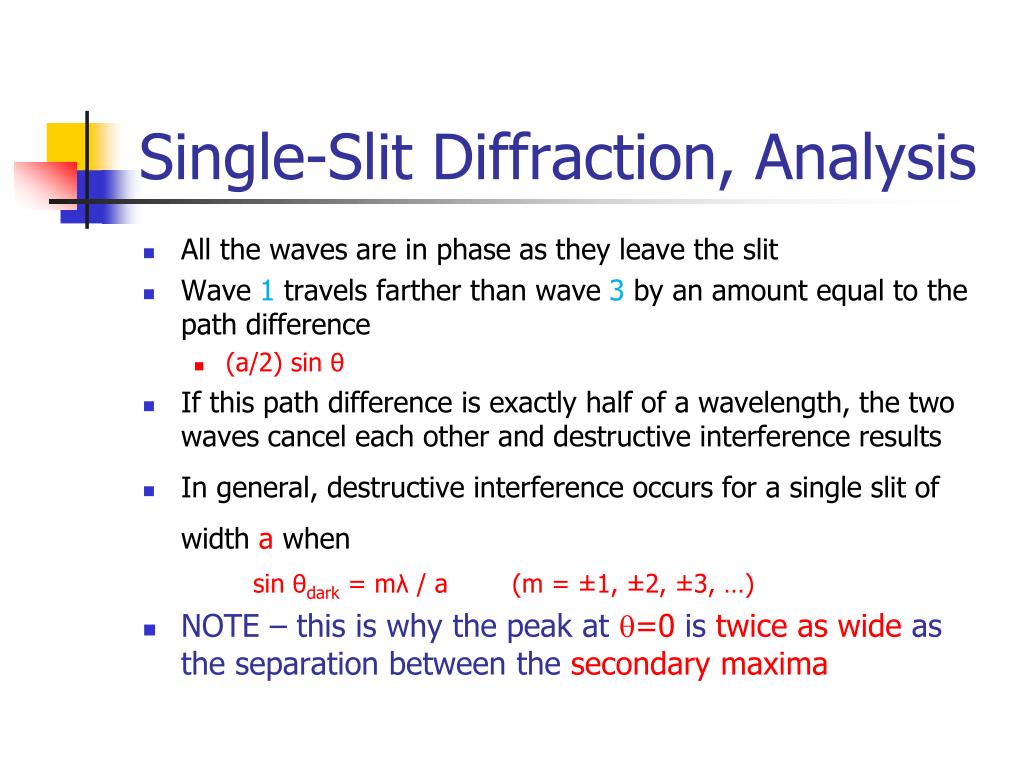
#Formula for single slit diffraction minima plus
If the distance to each source is an integer plus one half of a wavelength, there will be complete destructive interference. If the distance to each of the simple sources differs by an integer number of wavelengths, all the wavelets will be in phase, resulting in constructive interference. That is, at each point in space we must determine the distance to each of the simple sources on the incoming wavefront. Thus in order to determine the pattern produced by diffraction, the phase and the amplitude of each of the wavelets is calculated. Numerical approximations may be used, including the Fresnel and Fraunhofer approximations.ĭiffraction of a scalar wave passing through a 1-wavelength-wide slit Diffraction of a scalar wave passing through a 4-wavelength-wide slit General diffraction īecause diffraction is the result of addition of all waves (of given wavelength) along all unobstructed paths, the usual procedure is to consider the contribution of an infinitesimally small neighborhood around a certain path (this contribution is usually called a wavelet) and then integrate over all paths (= add all wavelets) from the source to the detector (or given point on a screen). Such treatments are applied to a wave passing through one or more slits whose width is specified as a proportion of the wavelength. For broader coverage of this topic, see Diffraction.ĭiffraction processes affecting waves are amenable to quantitative description and analysis.


 0 kommentar(er)
0 kommentar(er)
